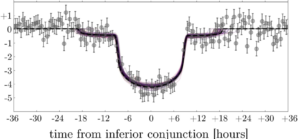
Kepler-1625 b’s transit as seen by Kepler, with the putative moon’s transit appearing as “ears” on either side. From Teachey et al. (2018a).

Last week, Alex Teachey and Dave Kipping of Columbia University presented impressive evidence for what would be the very first known moon orbiting an exoplanet.

The planet, Kepler-1625 b, is likely more massive than Jupiter but in an Earth-like orbit around an old (9 billion years old) but otherwise Sun-like star. Discovered in 2016 among data from the Kepler Mission, the planet was subjected to an intense analysis by Teachey and Kipping as part of the Hunt for Exomoons with Kepler program. In spite of their persistence, Teachey and Kipping found only hints of a moonshadow accompanying the planet’s distinct transit signal.

Hoping to corroborate their putative moon, they applied for and received 40-hours to observe the system with the Hubble Space Telescope (HST) and look for more lunar transits. In these data, Teachey and Kipping found even more convincing evidence for a moon.

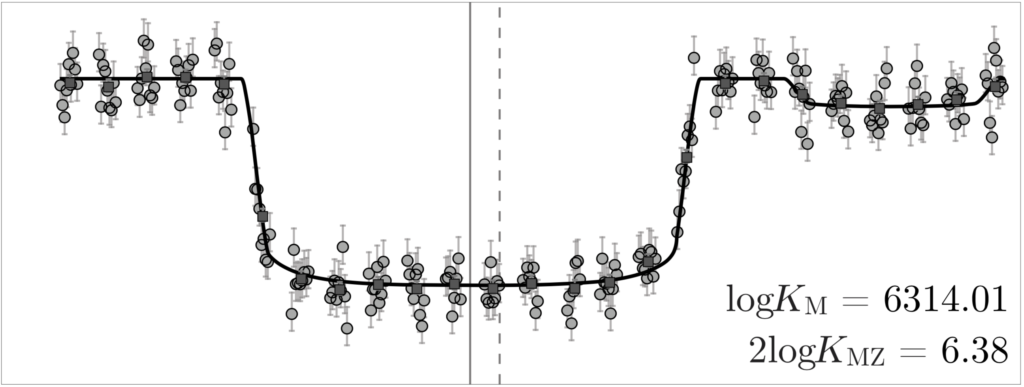
Kepler-1625 b’s and the putative moon’s transits as seen by HST. From Teachey et al. (2018b).

In the figure above, the little dip just to the right of the bigger dip (the planet’s transit) shows every sign of being the shadow of an exomoon circling a star about 7000 lightyears from Earth. Look around at how lucky we are to be alive right now.

Because of the extraordinary magnitude of their claim, Teachey and Kipping peppered their paper with lots of caveats, extending even to their paper’s title (“evidence for an exomoon”, not “we found a large exomoon”).

On top of that, they deployed an flotilla of statistical tests to argue in favor of the exomoon interpretation. One test in particular figures prominently in their analysis – the Bayes factor.

In this context, this ominous-sounding number is a measure of how much more likely one scientific model is over another, given a dataset. For instance, if you found your dog guiltily hiding from a mess in your house (your dataset), you would conclude there is a higher probability your dog made the mess (one scientific model) than a ghost did (another model).

A portrait of someone who may be (but probably isn’t) Thomas Bayes.

The Bayes factor derives from work by the Rev. Thomas Bayes, a minister living in Georgian England, who developed a method to infer the underlying probability for a particular experimental outcome, given results from several actual experiments.

Later, the scientist Simon-Pierre Laplace developed Bayes’ work into a more general theory of inference that he hoped could be used, for example, by juries to judge the guilt or innocence of a defendant.

Nowadays, Bayesian inference shows up everywhere, from analyses of climate change to estimates of the frequency of orange Reese’s pieces. It’s even possible that our brains are natively wired as Bayesian-inference machines.

And so in deciding whether they’d found an exomoon, Teachey and Kipping compared the probability that their Hubble data arose from a model including a lunar transit (as well as gravitational tugs between a planet and moon) to the probability the data showed a lone transiting planet.

Although, as they caution, these probability estimates can’t account for everything, they find the planet-moon model is 400,000 times more probable than the planet-only model.

As always, more data are needed to corroborate this fantastic result, but if it holds up, Kepler-1625 would be a system with one super-sized Jupiter-like planet accompanied by a Neptune-sized moon which orbits at a distance of about 300,000 km, not too different from our own moon’s distance.

Very shortly after Teachey and Kipping’s work was published, Kollmeier and Raymond explored the question of whether this monster moon could have its own moon and found that even a moon as large as Ceres could remain stable.

This result immediately prompted a more pressing question: should we call such a body a “sub-moon” or “moonmoon”?